
Besides, PCA has been used to quantify the contribution of the numerical noise of the algorithm and to identify the effect of artifacts related with the matching of the computational grid and the inner geometry of the photonic crystal.

Some other modes, contributing with an almost negligible amount to the total variance of the original data, are also revealed by the method. Finally, further properties such as the emission characteristics of a dipole near the crystal can be obtained from the Green’s tensor (4345). (FDTD) methods on a simulated nite-size pho-tonic crystal slab. The capability of PCA to produce spatial structures, or maps, associated with temporal evolutions has made possible the calculation of the modulus and phase of the modes existing in the photonic crystal. photonic crystal slab, where a periodic spatial modulation in. PCA has revealed and quantified the importance of the modes appearing in the photonic crystals. The Principal Component Analysis (PCA) has been applied here to a sequence of images corresponding to the electromagnetic fields obtained from the FDTD simulations. The definition of the modes associated with them is of interest for the study of the capabilities of photonic crystal devices.
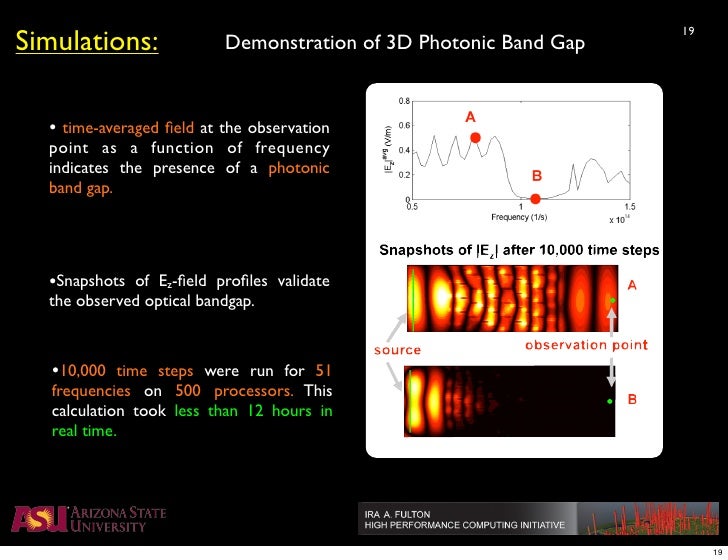
FDTD algorithms are being used as a numeric tool for the analysis of photonic crystals. Band-gaps, complex envelope (CE), locally one-dimensional finite-difference time-domain (LOD-FDTD) method, plasma photonic crystal (PPC) Abstract In this paper, a complex-envelope (CE) scheme is introduced into the locally one-dimensional finite-difference time-domain (LOD-FDTD) method for the band-gap analysis of the plasma photonic crystal (PPC).


 0 kommentar(er)
0 kommentar(er)
